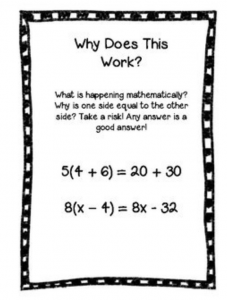
One of the biggest mistakes we make as teachers is making generalizations about math and teaching our students about them. For instance, I have had kids tell me, “I know we are going to multiply in this problem because the answers are bigger”, or “I know we divide because the answer is smaller” or when students say “I have to always put the bigger number on top when we subtract” These statements have been taught to these students throughout their years.
In some instances these are correct statements, but they are generalizations that are not always true. Later on they will subtract a greater number from a smaller number resulting in a negative number. In my case I saw all of these generalizations when I taught sixth grade. As their teacher, I had to go back and change their thinking and prove to them that the generalizations were not always true and show examples as to when they were not true.
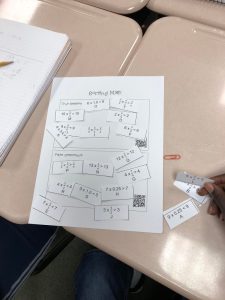
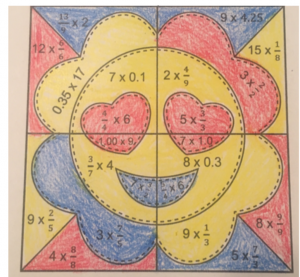
Multiplying fractions was one of the generalizations I had to conquer. In Texas, there is a standard that explicitly states for students to compare the product to the factors. This was a standard that I spent some time on even though it was a supporting standard. I feel like the supporting standards are just as important as the readiness standards because they lay the foundation for deeper, rigorous, and more meaningful operations. I prepared an activity that allowed students to activate prior knowledge (from fifth grade) to construct new meaning and form a new generalization about multiplying and finding the products.
In fifth grade, students multiplied whole numbers by fractions and vice versa, using models and repeated addition. This laid the foundation for what the sixth graders would be exploring. Students really took the time to analyze how the factors were related to the product.
* For instance, a whole number multiplied by a fraction less than one whole will result in a product that is less than the whole number factor used in the original problem. 5 x 1/4 < 5
* On the other hand, a whole number multiplied by a fraction more than a whole, will result in a product that is greater than the whole number factor used in the original problem. 5 x 6/4 > 5
* Finally, a whole number multiplied by a fraction equal to one whole will result in a product congruent or equal to the whole number used in the original problem. 5 x 4/4 = 5
Allowing students to fully explore these outcomes and come up with their own generalizations, gives students ownership for their own learning. Students came up with their own predictions and then tested those predictions and were validated with the results.



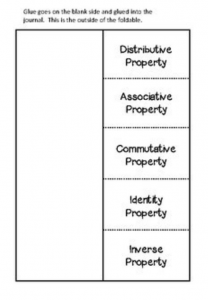
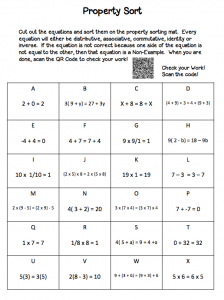

 Thank you for stopping by today! I hope you were inspired and you were able to find something new to try in your own classroom! If you didn’t find what you were looking for- please email me! I am happy to help!
Thank you for stopping by today! I hope you were inspired and you were able to find something new to try in your own classroom! If you didn’t find what you were looking for- please email me! I am happy to help!